Circuit Herbology
ex Grow circuits and processor components.
- Assign: Monday, 6 February
- Due: before class Monday, 13 February
- Collaboration: individual ex assignment policy, as defined by the syllabus and honor code.
- Submit: Bring a typed or neatly handwritten paper copy of this standard answer sheet to class.
- Relevant Reference:
Please download, print (2-sided), and write your answers on this standard sheet to help us grade and provide feedback to you faster.
1. Marauder’s Map (10 points)
| A | B | C | D | F(A,B,C,D) |
| 0 | 0 | 0 | 0 | 1 |
| 0 | 0 | 0 | 1 | 1 |
| 0 | 0 | 1 | 0 | 1 |
| 0 | 0 | 1 | 1 | 0 |
| 0 | 1 | 0 | 0 | 0 |
| 0 | 1 | 0 | 1 | 1 |
| 0 | 1 | 1 | 0 | 0 |
| 0 | 1 | 1 | 1 | 1 |
| 1 | 0 | 0 | 0 | 1 |
| 1 | 0 | 0 | 1 | 0 |
| 1 | 0 | 1 | 0 | 1 |
| 1 | 0 | 1 | 1 | 0 |
| 1 | 1 | 0 | 0 | 0 |
| 1 | 1 | 0 | 1 | 1 |
| 1 | 1 | 1 | 0 | 0 |
| 1 | 1 | 1 | 1 | 1 |
Draw a Karnaugh Map for the function F defined by the truth table at right. Use the Karnaugh Map to derive a minimal sum-of-products Boolean formula for F. Show any intermediate work. Review the reading on Karnaugh Maps carefully to understand the rules, especially with respect to wrapping boxes around.
2. Encoder a.k.a. the Undecoder? (8 points)
Draw a circuit to implement a 4-to-2 encoder using gates. An encoder is the opposite of a decoder. It takes 2n inputs and provides n outputs. Here, n = 2. Assume exactly one of the inputs carries a 1 and all others carry 0. The output should be an n-bit binary number giving the index of the input that is enabled. For example, if input 2 (in the range 0-3) is enabled and all others are disabled, the output should be 1 0.
3. Moving Staircases (8 points)
Draw a circuit to implement a switching network with two data inputs (A and B), two data outputs (C and D), and a control input (S). If S is 1, the network is in pass-through mode: output C should equal input A and output D should equal input B. If S is 0, the network is in crossing mode: C should equal B, and D should equal A. Use the most reasonable combinational building blocks or gates.
4. Value Judgments (24 points)
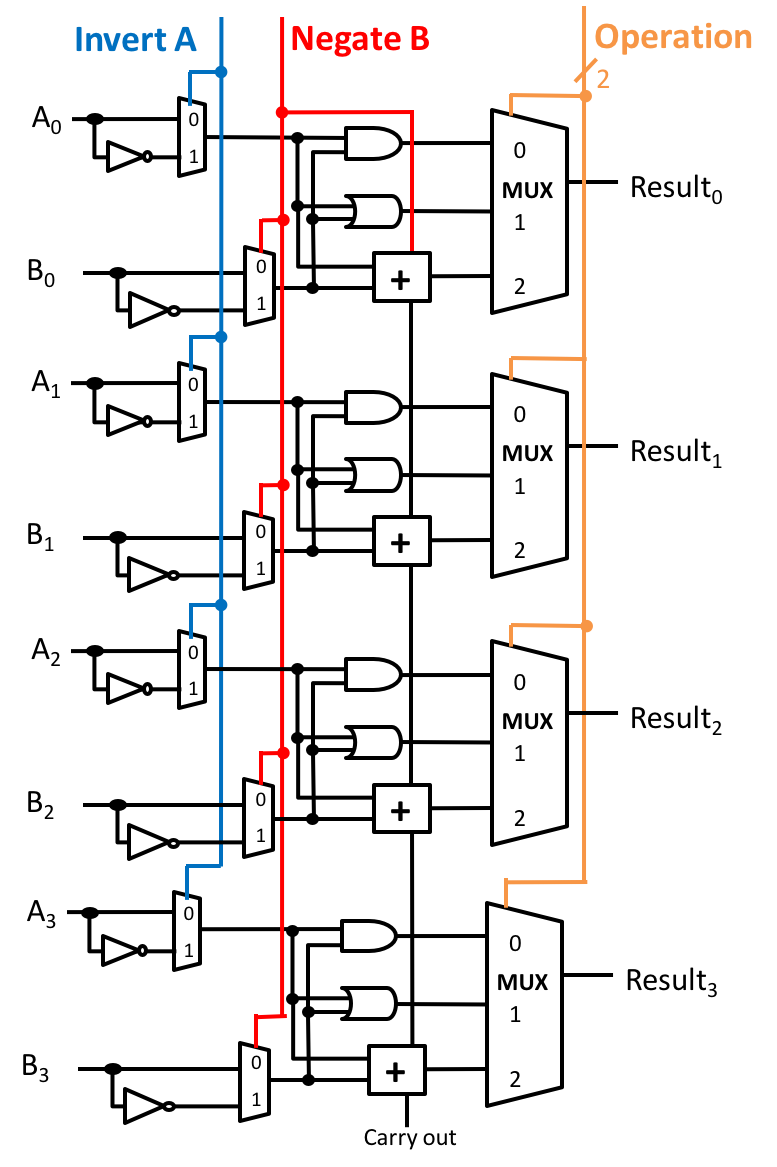
In class and lab we designed an n-bit ALU by connecting n 1-bit ALUs. In these exercises, you will improve the 4-bit version of our design.
Summary of our ALU design:
- The carry out from each bit is wired to the carry in of the next bit, if present.
- The full n-bit ALU has 4 lines of control input:
- Invert A controls the invert A inputs of all of the 1-bit ALUs.
- Negate B controls:
- the invert B inputs of all of the 1-bit ALUs; and
- the carry-in of the least significant bit ALU.
- Two bits of Operation ID control the Operation inputs of all of the 1-bit ALUs.
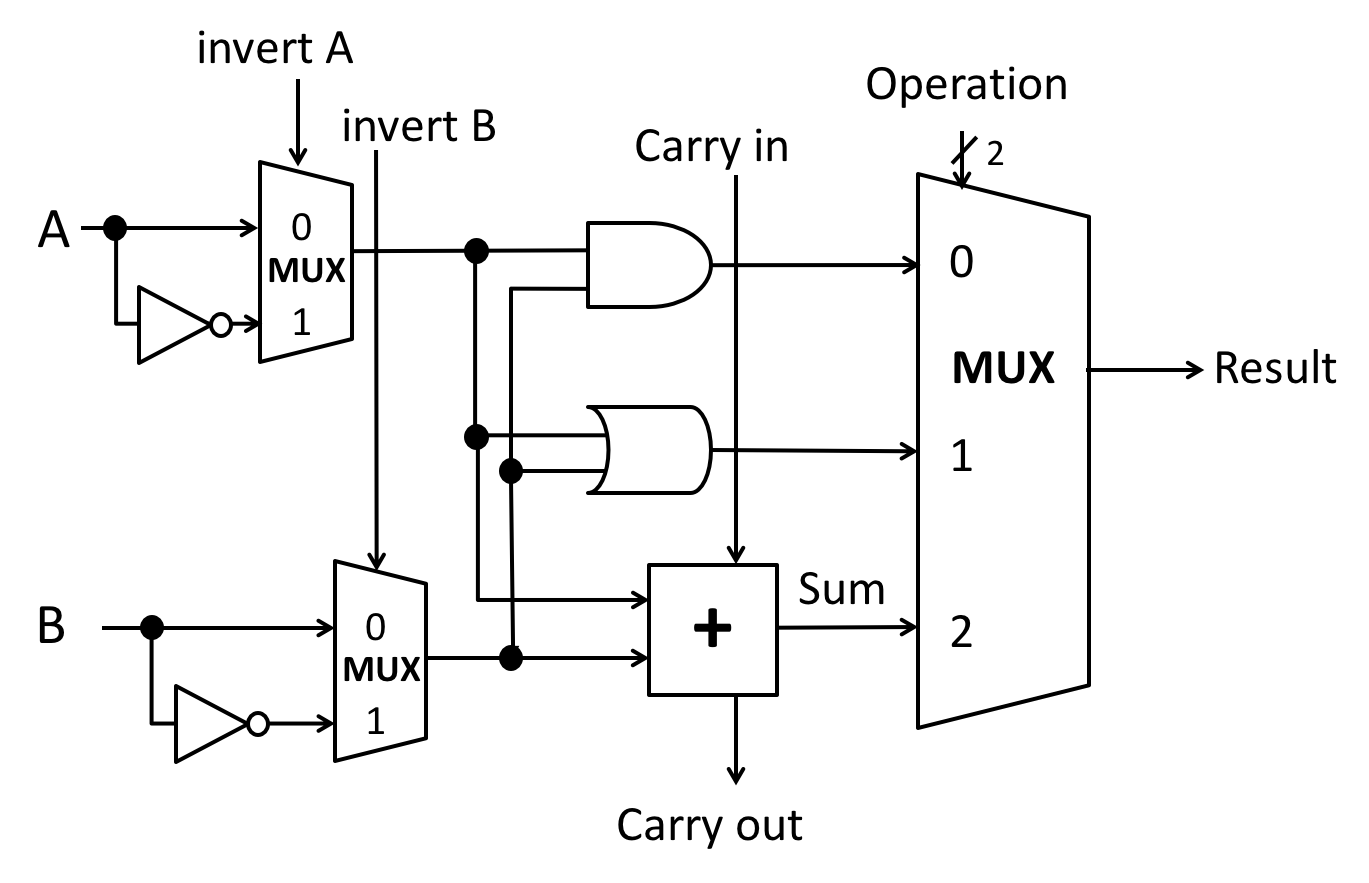
Rules for extending the ALU:
- Any additional logic may connect to any existing wires in the ALU and add gates or other building blocks as needed.
- Minimize the number of gates or other building blocks you add.
- Choose the simplest option and clearly label new outputs.
- Feel free to draw answers for separate exercises on separate diagrams or to combine them in one diagram, as you wish.
Exercises:
-
[2 points] Design extra 1-bit outputs from the n-bit ALU for the four conditions codes (i) Zero Flag, (ii) Sign Flag, (iii) Carry Flag, and (iv) Overflow Flag. Their definitions are given in the slides on ALUs. Using the provided sheet, draw and label the additional logic required for each condition code (flag), all on one ALU diagram. Feel free to reuse designs from your notes.
-
[6 points] Describe the output of the ALU when “Invert A” = 1, “Negate B” = 1, and “Operation ID” = 10.
- Write your answer as an arithmetic or Boolean/bitwise expression using A and B, e.g., Result = A & B or Result = 2A / B.
- Your expression must use either arithmetic operators or Boolean/bitwise operators. You may not mix them.
-
[8 points] Consider a less-than check for two’s-complement operands that computes A - B and returns a value based on the sign bit of the difference: 0 means A ≥ B, 1 means A < B.
- [1 point] Give a pair of values, A and B, where this approach correctly indicates A < B.
-
[1 point] Give a pair of values, A and B, where this approach incorrectly indicates A < B, even though actually, A ≥ B. Name the key effect that caused the answer to be incorrect.
- [6 points]
Redesign less-than so it works correctly for all pairs of
4-bit two’s-complement operands.
- Show how to control each of the 4 control lines of the full ALU.
- Using the provided sheet, draw minimal additional logic to compute the correct less-than result as a 1-bit output of the full ALU, in the style of the condition codes you implemented above, yielding 1 if A < B and 0 otherwise.
-
[6 points] Design a 1-bit equality test output for the ALU using minimal additional logic (but no additional XOR gates). Given inputs A and B, this output wire should carry 1 if A = B and 0 otherwise. Using the provided sheet, draw and label your design.
-
[2 points] Does the problem we encountered with less-than matter in your design of equality logic?
5. A Bit of Number Manipulation (10 points)
These problems will get you thinking in the style needed for the Bit Transfiguration assignment.
-
CSAPP3e Homework Problem 2.77.
(Summary:) Implement the C expression
x*kto multiply the Cintxby various constants,k, as listed. You may use only the C operators<<,-, and+, the variablex, and any literal numbers. Think about powers of 2. Each is possible with a small expression using only a few operators.x * 17x * -7x * 60x * -112
-
CSAPP3e Homework Problem 2.82.
(Summary:) For each of the following expressions, based on the given declarations, indicate whether the expression always yields 1 (for all possible
x,y,ux,uy, as established by these declarations). Indicate “yes” or “no.” You do not need to “describe the underlying mathematical principles” when indicating “yes.” Please provide counterexamples forxandyif indicating “no.”Declarations:
int x = ...; // any value int y = ...; // any value unsigned ux = (unsigned)x; unsigned uy = (unsigned)y;Expressions:
(x<y) == (-x>-y)((x+y)<<4) + y-x == 17*y+15*x̃x+ ̃y+1 == ̃(x+y)(ux-uy) == -(unsigned)(y-x)((x >> 2) << 2) <= x